
James Gregory (1638-1675)
In November 1638, Scottish mathematician and astronomer James Gregory was born. Gregory described an early practical design for the reflecting telescope – the Gregorian telescope – and made advances in trigonometry, discovering infinite series representations for several trigonometric functions.
James Gregory – Youth and Education
James Gregory was born at Drumoak, Aberdeenshire, UK, the youngest of the 3 children of John Gregory, an Episcopalian Church of Scotland minister. Initially he was educated at home by his mother, Janet Anderson, who endowed Gregory with his appetite for geometry, since her uncle Alexander Anderson has been a pupil and editor of French mathematician Francois Viète,[6] whose work on new algebra was an important step towards modern algebra. After his father’s death in 1651, Gregory was sent to Aberdeen Grammar School, and then to Marischal College, graduating in 1657.
The Gregorian Telescope
Gregory began to study optics and the construction of telescopes. Encouraged by his brother David, he wrote a book on the topic Optica Promota (The Advance of Optics), published in 1663. There, Gregory described his design for a reflecting telescope, the “Gregorian telescope“. A primary concave parabolic mirror converges the light to one focus of a concave ellipsoidal mirror. Reflection of light rays from its surface converge to the ellipsoid’s second focus which is behind the main mirror. There is a central hole in the main mirror through which the light passes and is brought to a focus by an eyepiece lens. The tube of the Gregorian telescope is thus shorter than the sum of the focal lengths of the two mirrors. His novel idea was to use both mirrors and lenses in his telescope. He showed that the combination would work more effectively than a telescope which used only mirrors or used only lenses.[1] The design pre-dates the first practical reflecting telescope, the Newtonian telescope, built by Sir Isaac Newton in 1668,[8] but was not successfully built until five years after Newton’s first reflecting telescope. Gregory also described the method for using the transit of Venus to measure the distance of the Earth from the Sun, which was later advocated by Edmund Halley [2] and adopted as the basis of the first effective measurement of the Astronomical Unit.

A Gregorian telescope of English manufacture circa 1735, owned by John Winthrop, on display at the Putnam Gallery in the Harvard Science Center
From Theory to Practice
Gregory’s aim was to find someone who could construct a telescope to the design set out in his book. John Collins advised him to seek the help of a leading optician by the name of Reive who, at Gregory’s request, tried to construct a parabolic mirror. His attempt did not satisfy Gregory who decided to give up the idea of having Reive construct the instrument. However, English natural philosopher Robert Hooke [3] learnt of Reive’s failed attempt at making the parabolic mirror and this would lead to a successful construction of the first Gregorian telescope around ten years later. In 1663 Gregory went to London, meeting John Collins and fellow Scot Robert Moray, one of the founders of the Royal Society. In 1664 he departed for the University of Padua, where he lived in the house of his countryman James Caddenhead, the professor of philosophy, and he was taught by Italian mathematician, philosopher, and Jesuat Stefano Angeli, working on the calculation of circular and parabolic surfaces by infinite convergent series.
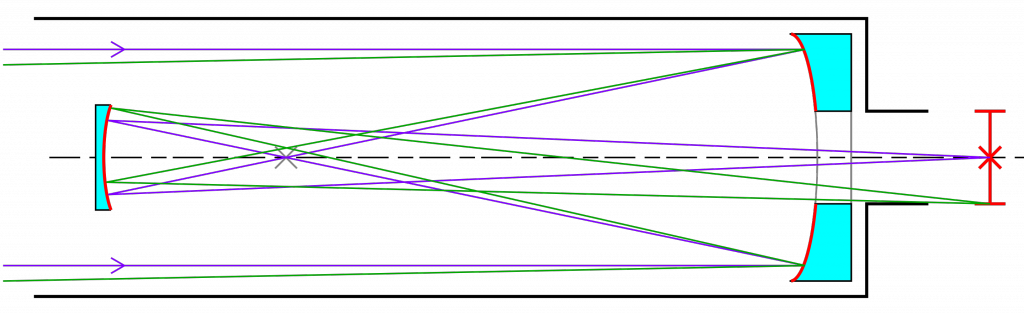
Diagram of a Gregorian reflecting telescope.
Contributions to Geometry
In 1667, Gregory issued his Vera Circuli et Hyperbolae Quadratura, in which he showed how the areas of the circle and hyperbola could be obtained in the form of infinite convergent series. This work contains a remarkable geometrical proposition to the effect that the ratio of the area of any arbitrary sector of a circle to that of the inscribed or circumscribed regular polygons is not expressible by a finite number of terms. Hence he inferred that the quadrature of the circle was impossible. In the same work he tried to estimate the unknown distances of bright stars. For Sirius he obtained 1.25 light years (instead of 8.6 LJ) by photometric comparison with the Sun and Jupiter, but for the first time the order of magnitude was correct.

James Gregory, Vera circuli et hyperbolae quadratura, 1667
Return to London
Upon his return to London in 1668 Gregory was elected a Fellow of the Royal Society, before travelling to St Andrews in late 1668 to take up his post as the first Regius Professor of Mathematics, a position created for him by Charles II, probably upon the request of Robert Moray. What is certain is that this summer he knew the Taylor series of sine and cosine as well as the tangent. The latter opened up new ways of calculating the circle. A year later he married in St Andrews Mary Jamesome who becomes the mother of two daughters and one son. Other important achievements followed in St. Andrews, such as the independent discovery of the Taylor formula in 1671, which Brook Taylor himself did not publish until 1715. Many of these works can only be found in letters to colleagues, as Gregory published very little after a plagiarism dispute with Christiaan Huygens. James Gregory also discovered the diffraction of light by bird feathers and investigated the diffraction patterns occurring in this process.
Last Years
About a year after assuming the Chair of Mathematics at Edinburgh, James Gregory suffered a stroke while viewing the moons of Jupiter with his students. He died a few days later at the age of 36. The Gregorian telescope design is rarely used today, as other types of reflecting telescopes are known to be more efficient for standard applications. Gregorian optics are also used in radio telescopes such as Arecibo, which features a “Gregorian dome”.[4]
Lynn Akin, Animate It – Gregorian Telescope, [10]
References and Further Reading:
- [1] O’Connor, John J.; Robertson, Edmund F., “James Gregory”, MacTutor History of Mathematics archive, University of St Andrews.
- [2] Edmond Halley and his famous Comet. SciHi blog, November 8, 2018.
- [3] Robert Hooke and his Micrographia, SciHi blog, July 18, 2013.
- [4] The Arecibo Radio Telescope – Looking for Extraterrestrial Signals, SciHi Blog, October 1, 2018.
- [5] Biography of James Gregory, at Britannica Online
- [6] Francois Viète and his New Algebra, SciHi Blog
- [7] Standing on the Shoulders of Giants – Sir Isaac Newton, SciHi Blog
- [8] James Gregory’s “Optica Promota” (English translation)
- [9] James Gregory at Wikidata
- [10] Lynn Akin, Animate It – Gregorian Telescope, History of Science Museum @ youtube
- [11] Timeline for James Gregory, via Wikidata





