On December 10, 1804, German mathematician Carl Gustav Jacob Jacobi was born. He made fundamental contributions to elliptic functions, dynamics, differential equations, and number theory.
“Any progress in the theory of partial differential equations must also bring about a progress in Mechanics.”
– Carl Jacobi, Vorlesungen über Dynamik [Lectures on Dynamics] (1842/3)
Carl Jacobi – A Child Prodigy
Carl Jacobi was the son of a banker and grew up in a rather wealthy jewish family. His brother, Moritz Jacobi became a famous physicist. Carl received his early education from his mother and entered the Gymnasium in Potsdam at about 12 years. However, his previous education was so good and he was so talented that the young Jacobi was put into the final class during his first year. This means that Carl Jacobi reached the necessary standard to enroll at a university when he was 12 years old. Unfortunately, the University of Berlin did not accept students below the age of 16 and Jacobi had to remain in the same class at the Gymnasium in Potsdam until the spring of 1821. [1]
At the University
At the university, he alternated for a long time between classical philology, in which he attended the lectures of August Boeckh, and mathematics, and also attended the philosophy lectures of Georg Wilhelm Friedrich Hegel and listened to history lectures. He learned mathematics primarily through self-study (e.g. Euler, Lagrange, Laplace), since the professors at the Berlin University at the time were, according to Jacobi, only mediocre mathematicians.[4] In 1824, he passed the senior teacher’s examination in Latin, Greek and mathematics. In 1825 he received his doctorate under Enno Dirksen (Disquisitiones Analyticae de Fractionibus Simplicibus), with Hegel sitting on the examination board. His habilitation (with an inaugural lecture on differential geometry) was completed in the winter semester of 1825/26
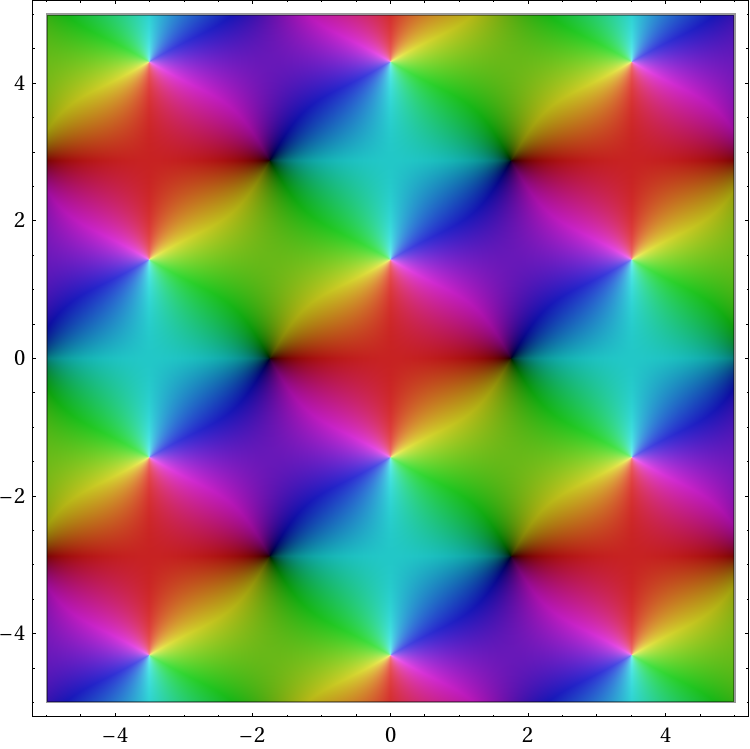
Complex graph of the Jacobi elliptic function sn u, with parameter m=√2
Image by Wikimedia User Fibonacci
The rights of citizenship and freedom in Germany for jews from 1812 was revoked in 1822 and all jews were officially taken from the civil services. Jacobi converted to Christianity in 1825 and became privatdozent. Jacobi was quite influenced by Gauss’ research on quadratic and biquadratic residues and studied cubic residues and informed Gauss of his discoveries, who was quite impressed. [3,5] By that time, Jacobi already made several major discoveries in the field of number theory and was privatdozent at Königsberg. He reformed university teaching there by founding a mathematics and physics seminar. The establishment of research seminars in mathematics was new at the time (but previously common in classical philology) and served as an example in Germany. In his lectures, too, he usually broke new ground and presented his own research. From 1827 he was an associate professor there and from 1829 a full professor.
Elliptic Functions
In this period, Jacobi also summarized his new ideas about elliptic functions and wrote a letter to Legendre, who was back then the leading expert on this topic. Legendre could have been mad or jealous that Jacobi (and also Abel) had made fundamental advances in his favorite topic, however, Legendre helped Jacobi to be promoted as associate professor in 1827. Legendre wrote a letter to Jacobi in this year:
It gives me great satisfaction to see two young mathematicians such as you and [Abel] cultivate with such success a branch of analysis which for such a long time has been my favourite topic of study but which had not been received in my own country as well as it deserves. By your works you place yourselves in the ranks of the best analysts of our era.
About two years later, Jacobi met Legendre as well as other mathematicians such as Fourier, Poisson, and Gauss, increasing his reputation in mathematics. The fundamental work on Jacobi’s theory of elliptic functions that impressed Legendre so much was based on four theta functions. Especially notable in this field is his paper Fundamenta nova theoria functionum ellipticarum, published in 1829. [1]
Later Years
In 1843, he gave up his lectures for health reasons (he suffered from diabetes) and, through the mediation of his friend Peter Gustav Lejeune Dirichlet and Alexander von Humboldt, received a grant from the Prussian state (Frederick William IV) to cure in Italy. He visited Lucca with his student Borchardt and Dirichlet and was in Rome in 1843/44, where the mathematicians Ludwig Schläfli and Jakob Steiner were also present at that time. In 1849, he fell into financial difficulties when he fell out of favor with the Prussian state because of his liberal political views in the revolution of 1848 (in which he was involved on the Republican side) (a position at the university Jacobi had sought was rejected, and the increase in his salary was cancelled in 1849), which, as with Gotthold Eisenstein, was alleviated by Alexander von Humboldt. In addition, his father’s bank had gone bankrupt some years earlier. He had to send his family to the cheaper Gotha in 1848. A call to the University of Vienna in 1850 improved his position vis-à-vis the Prussian state.
Carl Gustav Jacob Jacobi died in 1851 at the age of 46 years in Berlin from the consequences of a smallpox infection, having survived a flu shortly before.
Jeffrey Chasnov, Jacobi, Gauss-Seidel and SOR Methods | Lecture 66 | Numerical Methods for Engineers, [12]
References and Further Reading:
- [1] O’Connor, John J.; Robertson, Edmund F., “Carl Gustav Jacob Jacobi”, MacTutor History of Mathematics archive, University of St Andrews.
- [2] Gedächtnisrede auf Carl Jacobi gehalten in der Akademie der Wissenschaften 1852 [In German]
- [3] Carl Jacobi at Mathematics in Europe
- [4] Read Euler, he is the Master of us all…, SciHi Blog
- [5] Carl Friedrich Gauss – The Prince of Mathematicians, SciHi Blog
- [6] Adrien-Marie Legendre – Providing the Essential Analytical Tools for Mathematical Physics, SciHi Blog
- [7] . New International Encyclopedia. 1905.
- [8] Jacobi’s Vorlesungen über Dynamik
- [9] Carl Jacobi at zbMATH
- [10] Carl Jacobi at Mathematics Genealogy Project
- [11] Carl Jacobi at Wikidata
- [12] Jeffrey Chasnov, Jacobi, Gauss-Seidel and SOR Methods | Lecture 66 | Numerical Methods for Engineers, Jeffrey Chasnov @ youtube
- [13] Timeline for Carl Jabobi, via Wikidata






