
Giuseppe Peano (1858-1932)
On August 27, 1858, Italian mathematician and philosopher Giuseppe Peano was born. He is the author of over 200 books and papers, and is considered the founder of mathematical logic and set theory. The standard axiomatization of the natural numbers is named the Peano axioms in his honor. These axioms have been used nearly unchanged in a number of metamathematical investigations, including research into fundamental questions of consistency and completeness of number theory.
“Questions that pertain to the foundations of mathematics, although treated by many in recent times, still lack a satisfactory solution. Ambiguity of language is philosophy’s main source of problems. That is why it is of the utmost importance to examine attentively the very words we use.” (Giuseppe Peano, The Principles of Mathematics)
Peano Axioms
The need for formalism in arithmetic was not well appreciated until the work of German polymath and linguist Hermann Grassmann, who showed in the 1860s that many facts in arithmetic could be derived from more basic facts about the successor operation and induction. One should keep in mind that these days, the only axiomatic theory was Euclidean geometry, and the general notion of an abstract algebra had yet to be defined. In mathematics, an axiom is a premise or starting point of reasoning. As classically conceived, an axiom is a premise so evident as to be accepted as true without controversy. As used in modern logic, an axiom is simply a starting point for reasoning, a starting point for deducing and inferring other relative truths. The other way around, within the system they define, axioms (unless redundant) cannot be derived by principles of deduction, nor are they demonstrable by mathematical proofs, simply because they are starting points.
But, let’s get back to Peano’s axioms, before we take a look on Giuseppe Peano’s life. The Peano axioms contain three types of statements. The first axiom asserts the existence of at least one member of the set “number” (i.e. 0 is a number). The next four are general statements about equality (e.g., for every natural number x, x = x ). The next three axioms are first-order statements about natural numbers expressing the fundamental properties of the successor operation (i.e. the successor operation S(n):= n+1; the number ‘0’ is not the successor of any other natural number, etc.). The ninth, final axiom is a second order statement of the principle of mathematical induction over the natural numbers.
“1. 0 is a number. 2. The immediate successor of a number is also a number. 3. 0 is not the immediate successor of any number. 4. No two numbers have the same immediate successor. 5. Any property belonging to 0 and to the immediate successor of any number that also has that property belongs to all numbers.” (simplified version of the Peano Axioms)
Giuseppe Peano
Giuseppe Peano was born and raised on a farm at Spinetta, in the north of Italy. He attended the village school in Spinetta then he moved up to the school in Cuneo, making the 5km journey there and back on foot every day. He enrolled at the University of Turin in 1876, graduating as doctor of mathematics in 1880 with high honours. Peano joined the staff at the University of Turin in 1880, being appointed as assistant first to Enrico D’Ovidio, and then Angelo Genocchi, the Chair of Infinitesimal calculus. He published his first mathematical paper in 1880 and a further three papers the following year. Due to Genocchi’s poor health, Peano took over the teaching of the infinitesimal calculus course within 2 years.
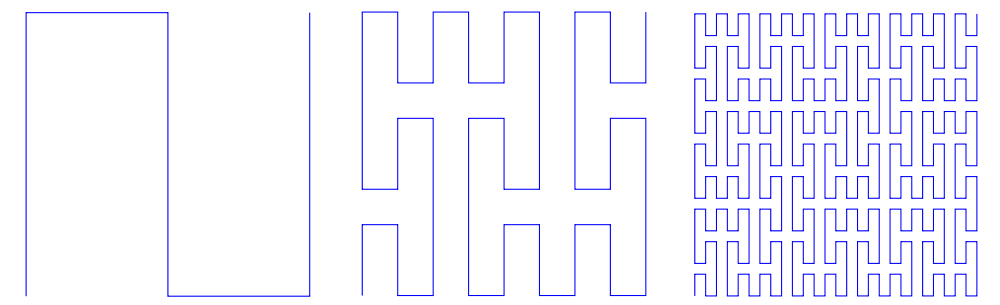
Three iterations of a Peano curve construction, whose limit
is a space-filling curve.
In 1886, he began teaching concurrently at the Royal Military Academy, and was promoted to Professor First Class in 1889. The next year, the University of Turin also granted him his full professorship. Peano’s famous space-filling curve appeared in 1890 as a counterexample. Hilbert, in 1891, described similar space-filling curves. It had been thought that such curves could not exist. Peano used his curve to show that a continuous curve cannot always be enclosed in an arbitrarily small region. This was an early example of what came to be known as a fractal. In 1889 Peano published his famous axioms, called Peano axioms, which defined the natural numbers in terms of sets. Peano had a great skill in seeing that theorems were incorrect by spotting exceptions. But, other mathematicians were not so happy to have these errors pointed out. In 1897, the first International Congress of Mathematicians was held in Zürich. Peano was a key participant, presenting a paper on mathematical logic. Paris was the venue for the Second International Congress of Mathematicians in 1900. The conference was preceded by the First International Conference of Philosophy where Peano was a member of the patronage committee. He presented a paper which posed the question of correctly formed definitions in mathematics, i.e. “how do you define a definition?“. This became one of Peano’s main philosophical interests for the rest of his life. At the conference Peano met Bertrand Russell, who was so struck by Peano’s innovative logical symbols that he left the conference and returned home to study Peano’s texts. Giuseppe Peano continued teaching at Turin University until the day before he died in 1932.
Peano’s Legacy
Peano’s Axioms led to a sequence of important discoveries in mathematics. When the Peano axioms were first proposed, Bertrand Russell [5] and others agreed that these axioms implicitly defined what we mean by a “natural number“. Henri Poincaré was more cautious, saying they only defined natural numbers if they were consistent; if there is a proof that starts from just these axioms and derives a contradiction such as 0 = 1, then the axioms are inconsistent, and don’t define anything. In 1900, David Hilbert [6] posed the problem of proving their consistency using only finite methods as the second of his twenty-three problems. In 1931, Kurt Gödel [11] proved his famous second incompleteness theorem, which shows that such a consistency proof cannot be formalized within Peano arithmetic itself.
Taylor Dupuy, Fundamentals of Mathematics – Lecture 11: Dedekind-Peano Axioms and More Induction, [8]
References and Further Reading:
- [1] O’Connor, John J.; Robertson, Edmund F., “Giuseppe Peano”, MacTutor History of Mathematics archive, University of St Andrews.
- [2] Peano’s curves at Cut the Knot
- [3] Hubert Kennedy: Twelve Articles on Giuseppe Peano
- [4] Giuseppe Peano at Princeton
- [5] The time you enjoy wasting is not wasted time – Bertrand Russell, Logician and Pacifist, SciHi Blog
- [6] David Hilbert’s 23 Problems, SciHi Blog
- [7] Giuseppe Peano at Wikidata
- [8] Taylor Dupuy, Fundamentals of Mathematics – Lecture 11: Dedekind-Peano Axioms and More Induction, Taylor Dupuy @ youtube
- [9] Works by or about Giuseppe Peano at Internet Archive
- [10] Giuseppe Peano at the Mathematics Genealogy Project
- [11] Giusepe Peano at zbMATH
- [12] Kurt Gödel Shaking the Very Foundations of Mathematics, SciHi Blog
- [13] Kennedy, Hubert C., 1980. Peano: Life and Works of Giuseppe Peano. Reidel. Biography with complete bibliography (p. 195–209).
- [14] Timeline of Guiseppe Peano via Wikidata





